Finding the unit digit of a large number’s cube might seem daunting at first, but it’s actually quite simple. If you’re looking to find the digit at the unit place of the cube of 5321, this guide will break down the process step-by-step, making it easy for anyone to understand and apply. No need for complex calculations or advanced math knowledge!
Understanding Unit Digits and Cubes
The unit digit of any number is simply the last digit. When we cube a number, we multiply it by itself three times. The trick to finding the unit digit of a cube is to focus only on the unit digit of the original number. Let’s explore this concept with a few examples.
Focusing on the Unit Digit
Consider the number 5321. The unit digit is 1. Now, let’s cube the unit digit: 1 x 1 x 1 = 1. Therefore, the unit digit of 5321 cubed is 1. It’s that straightforward!
Let’s try another example. Consider the number 74. The unit digit is 4. Cubing the unit digit: 4 x 4 x 4 = 64. The unit digit of 64 is 4. So, the unit digit of 74 cubed is 4.
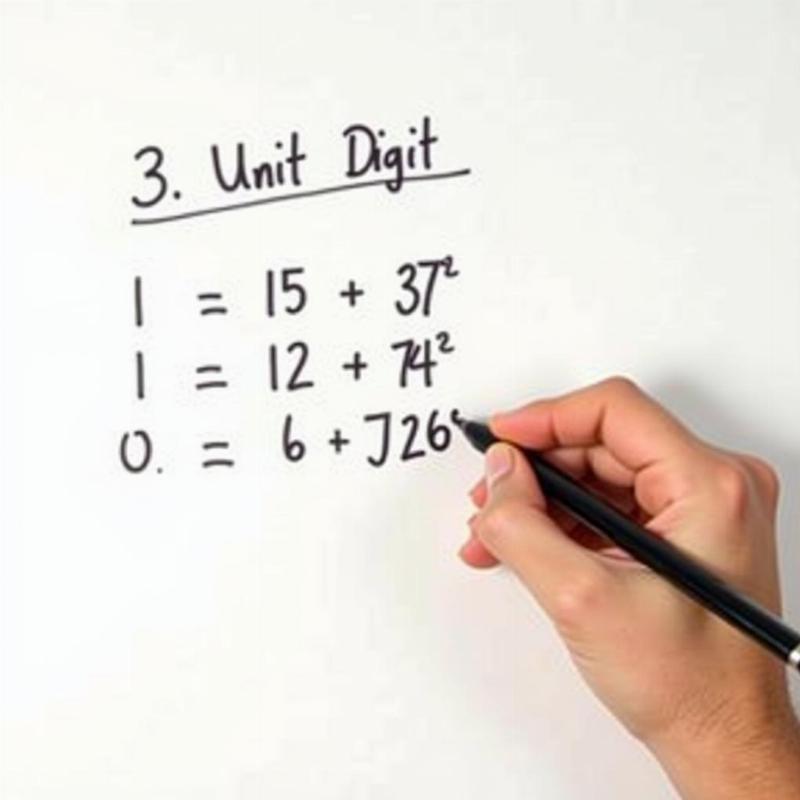Calculating the Unit Digit of a Cube
Applying the Concept to 5321
As we saw earlier, the unit digit of 5321 is 1. Cubing 1 (1 x 1 x 1) gives us 1. Therefore, the unit digit at the unit place of the cube of 5321 is 1.
Why Does This Method Work?
When multiplying numbers, the unit digit of the product is determined solely by the unit digits of the numbers being multiplied. The other digits influence the tens, hundreds, and so on, but not the unit place.
A Visual Explanation
Imagine multiplying 5321 by itself three times. You’ll notice that only the unit digit (1) contributes to the final unit digit of the result.
Practical Applications
Knowing this trick can be helpful in various scenarios, from quick mental math calculations to solving more complex mathematical problems. It also allows you to quickly verify the unit digit of a cubed number without performing the entire calculation, saving you time and effort.
Conclusion
Finding the digit at the unit place of the cube of 5321 is easy once you understand the underlying principle. By focusing on the unit digit of the original number and cubing it, you can quickly determine the unit digit of the final result, which in this case is 1. This simple method can be applied to any number, making it a valuable tool for anyone working with numbers.
FAQ
- What is a unit digit? The unit digit is the rightmost digit in a number.
- What does it mean to cube a number? Cubing a number means multiplying it by itself three times.
- Does this method work for all numbers? Yes, this method works for finding the unit digit of the cube of any whole number.
- Why is it important to focus only on the unit digit? Because the other digits don’t affect the unit digit of the final result when cubing.
- Can this method be applied to other powers like squares or fourth powers? Yes, a similar principle applies to other powers, though the calculations for the unit digits will be different.
- What are some real-world applications of this concept? This concept can be used for quick mental math, verifying calculations, and in certain competitive exams.
- Where can I learn more about number theory concepts like this? Many online resources and textbooks cover unit digits and related number theory topics.
PlaTovi, your trusted travel companion, offers comprehensive travel solutions, from customized tour packages to flight and hotel bookings. Whether you’re planning a traditional sightseeing tour with meals and shopping or looking for assistance with visas and other travel documents, PlaTovi can help. Contact us today to learn more about how we can make your travel dreams a reality! Email: [email protected], Phone: +91 22-2517-3581. We look forward to hearing from you!